Take our GED math practice test and see how you do. Our GED math diagnostic exam consists of 20 total questions on various math topics and concepts.
Click on the “Start Exam” button below to begin.
GED Math Diagnostic Pretest
Exam Summary
0 of 20 Questions completed
Questions:
Information
You have already completed the exam before. Hence you can not start it again.
Exam is loading…
You must sign in or sign up to start the exam.
You must first complete the following:
Results
Results
Time has elapsed
You have reached 0 of 0 point(s), (0)
Earned Point(s): 0 of 0, (0)
0 Essay(s) Pending (Possible Point(s): 0)
Categories
- GED Basic Algebra 0%
- GED Basic Math 0%
- GED Geometry 0%
- GED Graphs and Functions 0%
-
Keep Studying: We recommend that you keep studying. Your score is indicative of someone who should continue to brush up on GED math. Take some of our full-length practice exams.
-
Almost There: We recommend that you keep studying. You may be able to pass the GED math exam right now, but it will be close. Take some of our full-length practice exams.
-
You Are Ready: We feel you are ready for the GED math exam. To be safe, you may want to take one of our full-length exams to ensure you are fully prepared.
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- 19
- 20
-
Question 1 of 20
1. Question
CorrectIncorrect -
Question 2 of 20
2. Question
CorrectIncorrect -
Question 3 of 20
3. Question
CorrectIncorrect -
Question 4 of 20
4. Question
CorrectIncorrect -
Question 5 of 20
5. Question
CorrectIncorrect -
Question 6 of 20
6. Question
Sort elements
- 14.48
- 10.95
- 9.3
- 12.6
MeanCorrect Incorrect Correct answerMedianCorrect Incorrect Correct answerModeCorrect Incorrect Correct answerNot UsedCorrect Incorrect Correct answerCorrectIncorrect -
Question 7 of 20
7. Question
CorrectIncorrect -
Question 8 of 20
8. Question
CorrectIncorrect -
Question 9 of 20
9. Question
CorrectIncorrect -
Question 10 of 20
10. Question
CorrectIncorrect -
Question 11 of 20
11. Question
CorrectIncorrect -
Question 12 of 20
12. Question
CorrectIncorrect -
Question 13 of 20
13. Question
CorrectIncorrect -
Question 14 of 20
14. Question
CorrectIncorrect -
Question 15 of 20
15. Question
CorrectIncorrect -
Question 16 of 20
16. Question
CorrectIncorrect -
Question 17 of 20
17. Question
CorrectIncorrect -
Question 18 of 20
18. Question
CorrectIncorrect -
Question 19 of 20
19. Question
CorrectIncorrect -
Question 20 of 20
20. Question
CorrectIncorrect
Ready for All GED Subjects?
Take our comprehensive diagnostic exam that covers all 4 subjects of the GED. Instantly find out if you are ready for the exam.
GED Math Overview
The GED math section is 1 of 4 total sections on the GED exam. In order to receive your GED certificate, you will need to pass all 4 sections of the exam.
You can expect the following on the GED math portion of the exam:
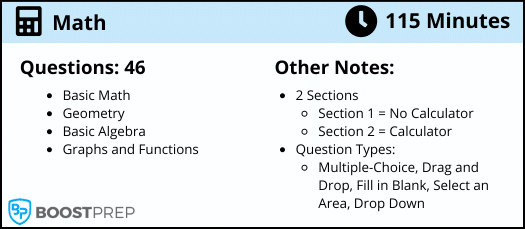
- Number of Sections: 2
- Number of Questions: 46
- Time Limit: 115 Minutes
Questions on this section of the exam will be broken into 4 main categories:
- Basic Math
- Geometry
- Basic Algebra
- Graphs and Functions
If you would like to practice for other sections of the exam, answer more of our GED practice questions.
GED Math FAQs
Yes, you will be able to use a calculator. However, there will be 1 short section in which you will not be able to use a calculator.
There will be about 46 total questions on the math portion of the exam. They will be split up between the 2 sections.
You will have a total of 115 minutes to take the math portion of the exam.
You will need to score a 145 or higher to pass GED math. Scoring higher than 145 can be beneficial as it may allow you to receive college credit or test out of courses in college.
Yes, you can retake the math section. However, the retake policy varies by state. Some states may require you to wait between retakes.
Our GED prep course covers all 4 subjects and includes 16 full-length exams. We cover every math concept you need to know and drill-down these concepts with over 900+ practice questions.